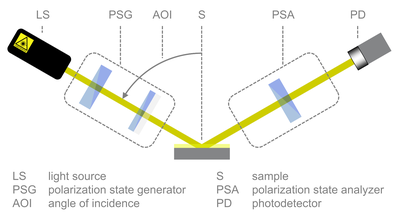
Ellipsometry is a non-destructive, optical technique of surface characterization. It probes a sample by shining in polarized electromagnetic radiation (“light”) at an oblique angle of incidence (AOI). The applied photon energies are typically in the range from the ultra-violet up to the infrared part of the electromagnetic spectrum. Ellipsometry detects and quantifies any change of the probing beam’s polarization ellipse – hence the name “ellipsometry” - that may occur upon its reflection from the sample surface (Fig.1).
An ellipsometric measurement is highly sensitive to any variations of the sample surface. In particular, ellipsometry excels at the characterization of transparent or semi-transparent thin-film coatings on flat, reflective sample surfaces. Thin-film layer thicknesses below 1 nm (i.e. mono-atomic or mono-molecular layers) may as well be measured as layers or layer-stacks with thicknesses of several microns.
What trick does ellipsometry play in order to reach its great accuracy and precision? It comes down to two key ingredients:
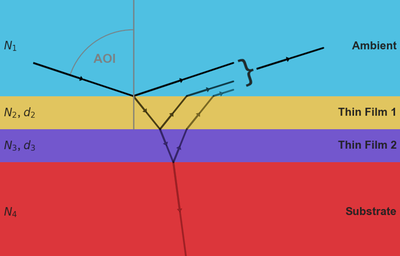
- Interference phenomena caused by multiple reflections within a transparent or semi-transparent thin-film layer result in a very strong dependence of the amplitude and phase of the reflected electromagnetic wave on the thin-film layer thickness and its refractive index (Fig.2).
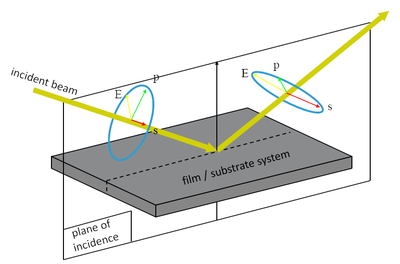
- Probing the sample with polarized light and at an oblique AOI gives access to the (relative) phase change for different states of incident polarization. This information would be lost at normal incidence or at the absence of polarization control (Fig.3).
While other optical metrology techniques such as reflectometry only use the intensity of the reflected beam (i.e. the amplitude of the reflected wave) for thin-film characterization, ellipsometry additionally gives access to the sample-induced (relative) phase shift of the probing wave. This additional phase-sensitivity finally gives rise to the excellent accuracy and sensitivity for thin-film layer characterization.
An ellipsometric measurement casts the sample’s polarization properties into two real numbers per probing photon energy, the so-called ellipsometric parameters Δ (“Delta”, phase information) and Ψ (“Psi”, amplitude information).
In order to understand the detailed meaning of these quantities, we need to introduce the mathematical description of the specular reflection of a polarized electromagnetic (EM) wave by a sample surface. In general, the amplitude and the phase of the incident EM wave change by the reflection. At an oblique AOI, however, the amount of these changes is different for a p-polarized and s-polarized wave (Fig.3), so we may write:
Ep,s and Ep,s' are complex numbers (i imaginary unit), which represent the electric-field amplitude and the phase of the p- and s-polarized EM waves prior to and after the reflection by the sample surface, respectively; rp,s and δp,s are the respective sample-induced amplitude and phase changes.
Finally, we may give the mathematical definition of the ellipsometric parameters Δ and Ψ:
Hence, Δ is the sample-induced phase difference for p- and s-polarized EM waves, whereas Ψ quantifies the ratio of the sample-induced amplitude changes of these waves.